0
In the classical paramagnetic theory of Langevin, pro- posed before the advent of quantum statistics, we assume a classical Hamiltonian, given by
where μi is the magnetic moment of a localized ion.
(a) Show that the canonical partition function of this system is given by
where dΩ is the elementary solid angle of integration.
Show that the magnetization (along the direction of the field) is given by
M = N ⟨µ cos θ⟩ = NµL (βµH) ,
where
L (x) = coth (x) - 1/X
is the Langevin function.
(b) Show that the susceptibility in zero field is given by the Curie law
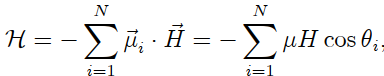
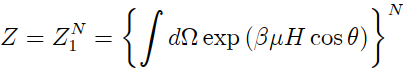
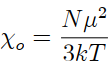
Physics
The magnetization is given by
where
Thus,
where L is the Langevin function.
need an explanation for this answer? contact us directly to get an explanation for this answer